Understanding the Order of Operations: PEMDAS
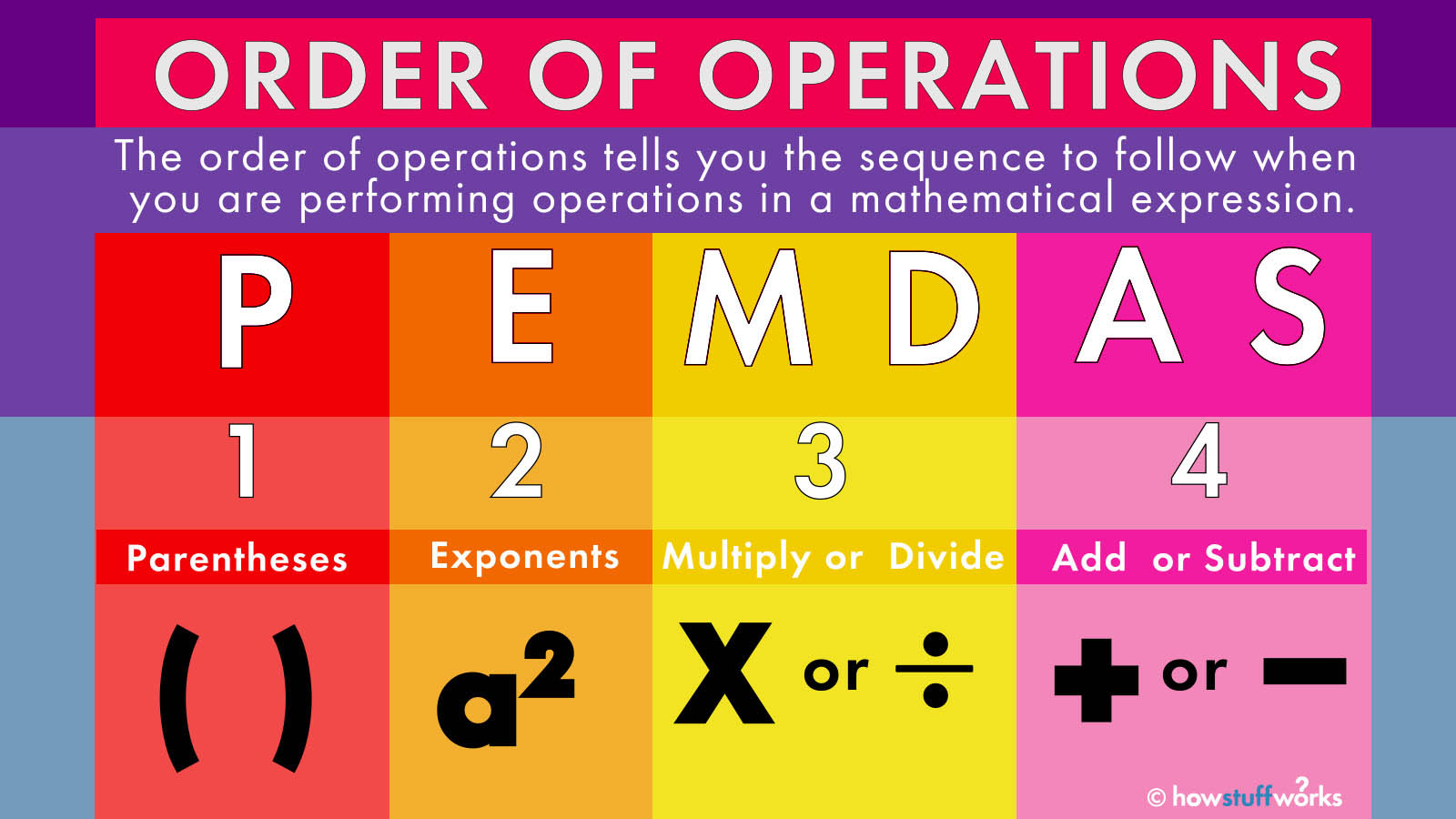
The Order of Operations is a fundamental concept in algebra and mathematics. It determines the sequence in which operations are performed to correctly solve expressions. The acronym PEMDAS stands for:
- Parentheses
- Exponents (including roots)
- Multiplication and Division (from left to right)
- Addition and Subtraction (from left to right)
Examples
Let's go through some examples to see how PEMDAS works in practice.
Example 1
Expression: 3 + (2 × 5)
Solution: According to PEMDAS:
- Evaluate inside parentheses: 2 × 5 = 10
- Then add: 3 + 10 = 13
Result: 13
Example 2
Expression: (4 + 3)² ÷ 7
Solution: Following PEMDAS:
- Evaluate inside parentheses: 4 + 3 = 7
- Then apply exponent: 7² = 49
- Divide: 49 ÷ 7 = 7
Result: 7
Example 3
Expression: 8 ÷ 2 × (2 + 2)
Solution: According to PEMDAS:
- Evaluate inside parentheses: 2 + 2 = 4
- Perform division and multiplication from left to right: 8 ÷ 2 = 4, then 4 × 4 = 16
Result: 16
Example 4
Expression: 5 + 6 × 3²
Solution: Following PEMDAS:
- Evaluate exponent: 3² = 9
- Perform multiplication: 6 × 9 = 54
- Add: 5 + 54 = 59
Result: 59
Example 5
Expression: (6 ÷ 2) × (1 + 2)
Solution: Following PEMDAS:
- Evaluate inside parentheses: 1 + 2 = 3
- Evaluate division: 6 ÷ 2 = 3
- Multiply: 3 × 3 = 9
Result: 9
Exercises
1. Evaluate: 7 + 2 × (3 + 2)²
2. Evaluate: (8 - 3) × 5 ÷ 5
3. Evaluate: 9 ÷ (3 + 3) × 2
Answers
1. Answer: 7 + 2 × 25 = 7 + 50 = 57
2. Answer: (8 - 3) × 5 ÷ 5 = 5 × 1 = 5
3. Answer: 9 ÷ 6 × 2 = 1.5 × 2 = 3
Lesson Summary
In this lesson, we've covered the Order of Operations using the acronym PEMDAS. We solved several examples and exercises to practice these concepts. Remember, correctly applying PEMDAS ensures that you get accurate results in mathematical expressions. Always work inside parentheses first, handle exponents next, then multiplication and division, and finally addition and subtraction from left to right.
For more help, check out Lunalearn 24 on Google, YouTube, or Facebook. If you need personal tutoring, visit our contact page to get in touch with us!
Understanding the Order of Operations: PEMDAS
The Order of Operations is a fundamental concept in algebra and mathematics. It determines the sequence in which operations are performed to correctly solve expressions. The acronym PEMDAS stands for:
- Parentheses
- Exponents (including roots)
- Multiplication and Division (from left to right)
- Addition and Subtraction (from left to right)
Examples
Let's go through some examples to see how PEMDAS works in practice.
Example 1
Expression: 3 + (2 × 5)
Solution: According to PEMDAS:
- Evaluate inside parentheses: 2 × 5 = 10
- Then add: 3 + 10 = 13
Result: 13
Example 2
Expression: (4 + 3)² ÷ 7
Solution: Following PEMDAS:
- Evaluate inside parentheses: 4 + 3 = 7
- Then apply exponent: 7² = 49
- Divide: 49 ÷ 7 = 7
Result: 7
Example 3
Expression: 8 ÷ 2 × (2 + 2)
Solution: According to PEMDAS:
- Evaluate inside parentheses: 2 + 2 = 4
- Perform division and multiplication from left to right: 8 ÷ 2 = 4, then 4 × 4 = 16
Result: 16
Example 4
Expression: 5 + 6 × 3²
Solution: Following PEMDAS:
- Evaluate exponent: 3² = 9
- Perform multiplication: 6 × 9 = 54
- Add: 5 + 54 = 59
Result: 59
Example 5
Expression: (6 ÷ 2) × (1 + 2)
Solution: Following PEMDAS:
- Evaluate inside parentheses: 1 + 2 = 3
- Evaluate division: 6 ÷ 2 = 3
- Multiply: 3 × 3 = 9
Result: 9
Exercises
1. Evaluate: 7 + 2 × (3 + 2)²
2. Evaluate: (8 - 3) × 5 ÷ 5
3. Evaluate: 9 ÷ (3 + 3) × 2
Answers
1. Answer: 7 + 2 × 25 = 7 + 50 = 57
2. Answer: (8 - 3) × 5 ÷ 5 = 5 × 1 = 5
3. Answer: 9 ÷ 6 × 2 = 1.5 × 2 = 3
Lesson Summary
In this lesson, we've covered the Order of Operations using the acronym PEMDAS. We solved several examples and exercises to practice these concepts. Remember, correctly applying PEMDAS ensures that you get accurate results in mathematical expressions. Always work inside parentheses first, handle exponents next, then multiplication and division, and finally addition and subtraction from left to right.
For more help, check out Lunalearn 24 on Google, YouTube, or Facebook. If you need personal tutoring, visit our contact page to get in touch with us!




0 Comments